The implications of removing repo assets from the leverage ratio
Published as part of the Macroprudential Bulletin 6, October 2018.
This article summarises the key findings from a counterfactual exercise where the effect of removing repo assets from the leverage ratio on banks’ default probabilities is considered. The findings suggest that granting such an exemption may have adverse effects on the stability of the financial system, even when measures are introduced to compensate for the decline in capital required by the leverage ratio framework. Increases in probabilities of default are mainly seen for larger banks which are more active in the repo market. Moreover, it is observed that the predictive power of the model improves when repo assets are included. Overall, the analysis in this article does not support a more lenient treatment of repo assets in the leverage ratio framework, e.g. by exempting them or allowing for more netting with repo liabilities or against high-quality government bonds.
1 Introduction
In the context of evaluating the impact of post-crisis regulatory reforms, concerns have been raised that the leverage ratio (LR) has had a negative impact on the functioning of repurchase agreement (repo) markets. To address these concerns, some stakeholders have suggested providing for a more lenient treatment of repo assets in the LR framework, e.g. by exempting them or allowing for more netting with repo liabilities or against high-quality government bonds. However, such a change in treatment could lead to the re-emergence of risks related to the build-up of excessive leverage and over-reliance on short-term wholesale funding in financial markets related to securities financing transactions and the re-use of collateral.[1]
This article intends to inform this debate by assessing the implications of such a regulatory change by considering two counterfactual exercises. Building on the framework developed in Acosta, Grill and Lang (2017)[2], first of all, the impact of exempting repo transactions from the LR framework on banks’ default probabilities is estimated. More specifically, in the counterfactual analysis it is assumed that banks will lower their capital by the amount that they had been required to hold against their repo asset before the change in treatment. At the same time, it is assumed that all banks will need to increase their LRs uniformly to compensate for this drop in capital required in the whole banking system.[3] Second, in a complementary exercise, it is analysed whether the change in regulatory treatment affects the predictive power of the LR for bank default.[4]
2 The impact of bank default probabilities
To assess the impact of banks’ leverage on their distress probabilities, following Acosta, Grill and Lang (2017) in a first exercise a panel logit model with time and country fixed effects is estimated for a sample of 500 European banks. On the basis of this model, bank default probabilities are predicted.
In the above equation, is the cumulative distribution function of the logistic distribution. The left-hand-side variable is the binary distress indicator for bank 𝑖 in year 𝑡 + 1; 𝑋𝑖,𝑡 and 𝑌𝑗,𝑡 are vectors of bank-specific and country-specific control variables (such as capital over risk-weighted assets, non-performing loans over total assets, pre-tax return over assets, loans over total assets, GDP, the unemployment rate, etc.); and and are time and country fixed effects respectively. is the leverage ratio measured as observed capital levels over total assets, i.e. over
The dataset for the empirical analysis consists of a large unbalanced panel of 500 EU banks for the period 2005-14. For the analysis, data from multiple sources are used: (1) bank-specific variables from SNL and Bankscope; (2) country-level macroeconomic variables from the ECB Statistical Data Warehouse; and (3) a unique collection of bank distress events that covers bankruptcies, defaults, liquidations, State aid cases and distressed mergers.[5]
In a second exercise, a two-step counterfactual analysis is conducted. In a scenario under which repo assets are exempted from the LR, first of all, banks’ new level of capital and total assets ( are re-estimated. More specifically, it is assumed that banks will lower their capital by exactly the amount they had been required to hold against their repo asset before the change in treatment. In other words, it is assumed that an exemption of repo assets would incentivise banks to reduce capital () to a new level (such that banks’ LRs are kept constant, i.e.:
It is also assumed that an exemption is likely to be accompanied by some form of compensatory measure to keep the overall level of capital in the banking sector constant. As a consequence, an exemption of repo assets in the LR treatment will be accompanied by a simultaneous increase in the minimum LR requirement to ensure that the total amount of capital in the system stays constant.[6] More specifically, it is assumed that this is achieved by a uniform increase in capital across all banks following the exemption of repo assets. Hence, to maintain a constant level of capital across the 500 banks in the sample, the average capital per bank has to increase by 6.8%.[7] In a second step, using these new levels of capital the individual bank default probabilities are re-estimated and then compared with the ones that were obtained without the policy change. The model in Equation (1) is re-estimated but now includes the new leverage ratio ( and the new distress probabilities.
Comparing the default probabilities in the two states (with and without a change in the treatment of repos in the LR framework) suggests that the asset-weighted median bank default probability increases by 7%. The asset-weighted median bank default probability is specified as the median default probability of all banks in the sample ordered by assets and not by magnitude of default probability.[8] The 7% increase is a significant one, which suggests that the exemption of repo assets from the LR may have adverse effects on the stability of the financial system, even when measures are introduced to compensate for the decline in capital required by the LR framework.
When focusing on the ten banks with the largest repo position in absolute terms, it is observed that for this set of banks the median default probability increases by 91%. Given the systemic relevance of these banks, an exemption of repo assets may be considered particularly harmful from a financial stability perspective. The effect is greater for these banks, because the repo assets are very asymmetrically distributed across the banks: while most banks only hold negligible amounts of repo assets, the set of large banks report a considerable part of the repo assets in the sample.
To get an idea of the economic magnitude of these changes, the 7% increase for all banks and the 91% increase for the ten largest banks are compared with changes in the median default probability for the year 2009. More specifically, the results are compared with the 2009 numbers since annual defaults in the sample peaked in 2009. Compared with pre-crisis averages[9], asset-weighted default probabilities increased by 250% in 2009. This implies that a change in default probabilities of 91% for big banks (following the exclusion of repo assets) represents more than one-third of the observed changes in median default probabilities between the pre-crisis period and the peak of systemic uncertainty in the post-crisis period.
In addition, the effect of exempting central bank reserves is also considered. An exemption of both balances with central banks and repo assets would lead to a decrease in total capital of 9% which is offset by uniformly increasing the amount of capital to compensate for this decrease. The results show that the median total asset-weighted default probability increases by 16%. Hence, considering the combination of exemptions of central bank reserves and repo assets more than doubles the effects observed for the repo exemption as some banks (in particular larger ones) significantly benefit from both changes in regulatory treatment.
Chart 1
The non-linear relationship between the leverage ratio and default probability
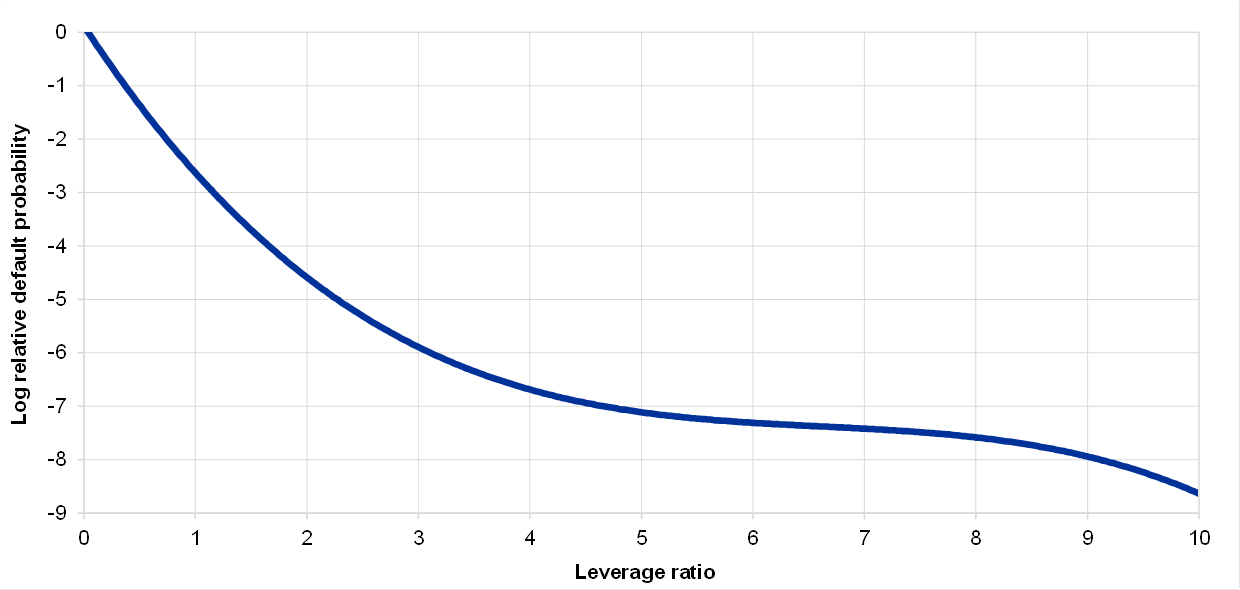
Source: Acosta Smith, J., Grill, M. and Lang, J.H., “The leverage ratio, risk-taking and bank stability”, Working Paper Series, No 2079, ECB, June 2017.
Notes: The log relative distress probability is equal to the log of the probability of distress divided by the probability of non-distress. Specifically, if the probability of distress is given by p, then it is equal to log(p/(1 − p)).
Chart 1 shows that the amplification effect is driven by a non-linear relationship between the LR and the default probability. When the LR decreases to a level of 3% from higher levels, the graph illustrates that the default probability changes proportionally more than for a change of the LR from 6% to 5%. The change in the regulatory treatment for both central bank reserves and repo assets pushes the banks’ original leverage ratios further to the left as they can significantly reduce their capital and may increase the probability of distress substantially. It is noteworthy that this effect particularly holds for some larger banks that had low LRs to begin with and that benefit most from the exemptions.
3 Implications for the predictive power of the leverage ratio
In a second analysis, it is assessed whether the change in regulatory treatment affects the predictive power of the LR for bank default. Using the framework developed in Acosta, Grill and Lang (2017), the goodness of fit of the model for alternative measures of the LR is examined. More specifically, the ability of diverse measures to explain variation in probabilities of default and the in-sample fit are compared.
Table 1
Predictive power of different models

*LR without repo is not significant at the 1% level.
Assessing the pseudo R², a measure of the quality of the different models, in Table 1 it can be observed that the model using the LR without repo is slightly less suited to fitting the data (second row).[10] The model in the bottom row of Table 1 separately accounts for repo assets/total assets. This highlights that repo assets are an important factor to assess the default risk of a given bank regardless of whether they are included indirectly through the LR or separately as an extra indicator. If we include repo assets/total assets separately, this even increases the model’s in-sample performance slightly.
Assessing the out-of-sample predictive power of the model using ROC curves, it can be observed that model performance is very similar for both specifications. ROC curves are a standard tool to evaluate and compare predictive models. Accuracy is measured by the area under the ROC curve, where broadly speaking an area of 1 represents a perfect test. For the analysis, the areas under the ROC curves are 0.913 for the model with an LR including repo positions and 0.912 for the model excluding repo positions. Overall, while the results are not very strong, we find that excluding repo assets slightly decreases the fit of the model.
4 Conclusion
Overall, the findings suggest that the exemption of repo assets (and central bank reserves) from the LR may have adverse effects on the stability of the financial system, even when measures are introduced to compensate for the decline in capital required by the LR framework. Against the background of a pronounced increase of median default probabilities for large systemically relevant banks, an exemption of repo assets could considerably weaken the LR for some banks and may have adverse effects from a financial stability perspective.
The analysis therefore does not support a more lenient treatment of repo assets in the LR framework, e.g. by exempting them or allowing for more netting with repo liabilities or against high-quality government bonds. As the analysis does not consider the potential negative consequences of increased repo activity following the exemption of repo assets from the LR exposure measure, the estimate of a potential increase in default probability should be seen as a lower bound.
The results in this article complement previous findings[11] of an overall functioning repo market in the euro area, showing that regulatory reforms have not had a material unintended effect on the amount of euro area banks’ outstanding repo transactions. It should be borne in mind that the analysis in this article does not consider the negative consequences of increased repo activity following an exemption of repo assets from the LR exposure measure, including a potential further increase in default probability.
References
Acosta Smith, J., Grill, M. and Lang, J.H. (2017), “The leverage ratio, risk-taking and bank stability”, Working Paper Series, No 2079, European Central Bank, June.
Aikman, D., Galesic, M., Gigerenzer, G., Kapadia, S., Katsikopoulos, K., Kothiyal, A., Murphy, E. and Neumann, T. (2014), “Taking uncertainty seriously: simplicity versus complexity in financial regulation”, Bank of England Financial Stability Paper No 28, May.
Betz, F., Oprica, S., Peltonen, T.A. and Sarlin, P. (2014), “Predicting distress in European banks”, Journal of Banking & Finance, Vol. 45.
Financial Stability Board (2017), “Re-hypothecation and collateral re-use: Potential financial stability issues, market evolution and regulatory approaches”, January.
Grill, M., Jakovicka, J., Lambert, C., Nicoloso, P., Steininger, L. and Wedow, M. (2017), “Recent developments in euro area repo markets, regulatory reforms and their impact on repo market functioning”, Financial Stability Review, European Central Bank, November.
Schularick, M. and Taylor, A.M. (2012), “Credit Booms Gone Bust: Monetary Policy, Leverage Cycles, and Financial Crises, 1870-2008”, American Economic Review, Vol. 102(2), April.
The FSB Re-hypothecation and Re-use Expert Group also highlighted the LR as the main brake put in place after the crisis to address these concerns. See Financial Stability Board (2017), “Re-hypothecation and collateral re-use: Potential financial stability issues, market evolution and regulatory approaches”, January.
Acosta Smith, J., Grill, M. and Lang, J.H. (2017), “The leverage ratio, risk-taking and bank stability”, Working Paper Series, No 2079, ECB, June.
This could, for example, be achieved by uniformly increasing the minimum LR requirement as the Bank of England has done in the case of the exemption of central bank reserves. It should be noted that such a uniform increase may disproportionally affect banks not involved in repo business and may therefore cause additional costs.
It is important to note that the leverage ratio is a better predictor of bank default than the risk-based ratio; see, for example, Betz, F., Oprica, S., Peltonen, T.A. and Sarlin, P. (2014), “Predicting distress in European banks”, Journal of Banking & Finance, Vol. 45, and Aikman, D., Galesic, M., Gigerenzer, G., Kapadia, S., Katsikopoulos, K., Kothiyal, A., Murphy, E. and Neumann, T. (2014), “Taking uncertainty seriously: simplicity versus complexity in financial regulation”, Bank of England Financial Stability Paper No 28, May. In addition, Schularick, M. and Taylor, A.M. (2012), “Credit Booms Gone Bust: Monetary Policy, Leverage Cycles, and Financial Crises, 1870-2008”, American Economic Review, Vol. 102(2), April, highlight that excessive leverage contributes to financial instability.
Bank distress events are taken from Acosta, Grill and Lang (2017).
This could, for example, be achieved by uniformly increasing the minimum LR requirement as the Bank of England has done in the case of the exemption of central bank reserves.
This increase in capital translates into a change in the LR from 5.9 to 6.3 for the median bank.
This measure (instead of median default probability) is considered for two reasons. First, it is a more systemic measure since it gives more weight to banks with more assets. Second, larger banks tend to have larger shares of repo in terms of total assets and therefore benefit more from the reduction of capital required and are relatively less affected by the compensating increase in capital requirements. Calculating the median default probability would not show the increase above, since it would not account for the asymmetric holdings of repo assets per bank.
This is the average of the years 2005, 2006 and 2007 in the sample.
It should be noted that the leverage ratio also enters the model at lower levels of significance.
Grill, M., Jakovicka, J., Lambert, C., Nicoloso, P., Steininger, L. and Wedow, M. (2017), “Recent developments in euro area repo markets, regulatory reforms and their impact on repo market functioning”, Financial Stability Review, ECB, November.


